Back to the Lertap 5 home page.
(www.lertap.curtin.edu.au)
The
‘CHPQ’: Cognitive Holding Power Questionnaire
(Entered 4 September 2002.)
Professor John Stevenson of Griffith University has developed an instrument which is “…designed to measure the extent to which learning settings press students into different levels of thinking” (Stevenson, 1998; also see Stevenson & Evans, 1994). He has kindly made his work available for others to study and use; to see the complete CHPQ instrument, click here.
The CHPQ consists of 30 items, but only 27 are used in the actual scoring of the instrument. Thirteen of the items are scored to produce the FOCHP result, that is, the score for a student’s First Order Cognitive Holding Power. Fourteen other items are scored in order to derive the SOCHP result, or Second Order Cognitive Holding Power score.
The development of the CHPQ instrument provides a classical example for measurement classes, involving the assessment of the instrument’s reliability, validity, and factorial complexity. In terms of references for students, see Stevenson & Evans (1994) for a discussion of factor analyses, and Stevenson (1998) for respective reliability and validity work.
A click here will take you to a sample data set, an Excel workbook ready to work with Lertap. Thanks go to Ian Boyd of the Carlisle campus of TAFE for making this data set available.
You’ll see that the sample data were entered starting in the first column of the Data worksheet. The lines in the CCs sheet indicate which items belong to which of the two scales.
Responses to the CHPQ were kept anonymous. When staff at Carlisle processed the CHPQ returns from students, they wrote a simple sequential processing number at the top of each return. This number was recorded in the last column of the Data worksheet. Such numbers are useful when it becomes necessary to backtrack—for example, processing the data may reveal that someone answered “8” to one of the items, which is invalid and probably representative of a data processing error. If we can find the line with the 8 in the Data sheet, we can then find the corresponding ID by looking in the line’s last column, and this will take us back to the original returns. Once there we can easily determine what the 8 was meant to be.
To get results, keep the sample data set open, and get Excel to load the Lertap5.xls file. Then switch back to the sample data set, and use Lertap’s Run menu to Interpret CCs lines, followed by a run of Elmillon.
The data set may be processed with the (free) Student Version of Lertap by doing these things: inserting a new row after row 22 of the Data sheet, and inserting a new row after row 3 of the CCs sheet. Doing this will leave a blank row in each worksheet, and will cause Lertap to ignore subsequent rows; the Data sheet will appear to Lertap to have just 20 records, and Lertap will think there’s only one subtest. (These steps are necessary as the Student Version supports no more than 20 data records, and no more than one subtest. The subtest may not have more than 20 items. Click here to link out to a copy of the Carlisle data set which will work with the Student Version of Lertap 5.)
The results from the Carlisle TAFE students give subtest reliabilities lower than those reported by Stevenson in the literature. With all 32 students, Lertap reports an FOHCP reliability (coefficient alpha) of 0.64, with a figure of 0.75 for SOCHP. Stevenson (1998) found FOCHP alpha reliabilities of 0.82 to 0.86, and SOCHP figures of 0.77 to 0.87 “across a variety of courses in different colleges”.
In the Carlisle sample two items on the second subtest, FOHCP, have negative correlations; these items have served to lower the reliability estimate. Of course the Carlisle data set is a very small one, and these results in no way impugn the integrity of the CHPQ as a research instrument.
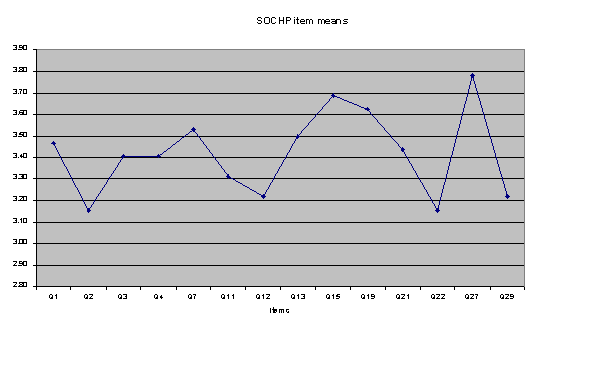
Some data analysis questions and activities to pursue: (1) what was the correlation between SOCHP and FOCHP scores in the Carlisle sample (have Lertap make a corresponding scatterplot)?; (2) what would the value of coefficient alpha have been for the FOCHP subtest if the items with negative correlations had been omitted?; (3) the graph below plots the item means for the SOCHP subtest—make a similar one for FOCHP.
References
The references
cited above are to be found on the Lertap website. If you’re connected to the Internet, click here to bring up the References page.
Professor
Stevenson may be reached by email. As
of September, 2002, his address for email correspondence was: j.stevenson@mailbox.gu.edu.au. His university’s website is found at www.gu.edu.au.